networks dynamical systems basics

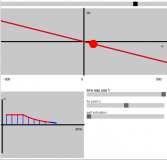
Processes happen in time. The mathematical tool to describe and model processes is called dynamical systems theory. Typical applications are climate models, models of chaotic behavior or physical simulations. Any system that evolves in time can be approximated with dynamical equations.
Here we consider a very basic equation \(\dot{u}=h (u+c_{fix})\). This equation describes the rate of change of a variable u, that could for example be the position of a ball, as a function of its on state.